Hi, how do solve this? Greg solved it but, I couldn’t get it. He solved it just by looking at the last 2 digits, how’s it possible? If there’s any division rule or shortcut, please do let me know.
You can look up divisibility rules.
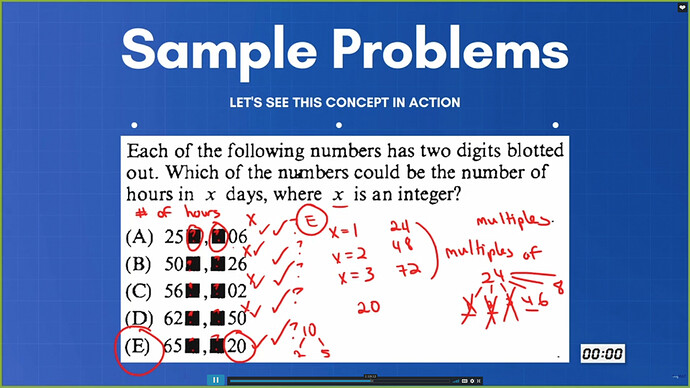
For 2 - all of them are even. (Your choices are even)
For 3 - sum of numbers are divisible by 3 (your numbers are blotted out, won’t help)
For 4 - if the last two digits are divisible by 4 the number is divisible by 4 (option E works)
Why 4 you may ask. Find the factors of 24 (1,2,3, 4 etc). Every multiple of 24 will also be divisible by the factors of 24. You tried 2, and 3. Didn’t help. So you then tried 4 and it worked. Hope that helped
OK thanks for the divisibility rules, I got those. But, this:
Why 4 you may ask. Find the factors of 24 (1,2,3, 4, etc). Every multiple of 24 will also be divisible by the factors of 24. You tried 2, and 3. Didn’t help. So you then tried 4 and it worked. Hope that helped
I am a bit confused here, if option (E) isn’t divisible by 3 (as 3 is the factor of 24) then how can it be divisible by 24? I’m sorry if it sounds noob, I think I’m good at maths but here I’m totally off.
I meant that you can’t do the divisibility test using 3 because you need to know all the numbers. But all of the answer choices have two blotted out numbers, so you can’t do the divisibility test.
If I said is 933 divisible by 3? You would say yes. If I said is 9_3 divisible by 3? You can’t guarantee it because the middle number could be anything
OK, then why option E? Option A isn’t divisible by 4 but by 3, and both are divisible by 2. If you don’t mind would you please explain the complete process in this question? I might be missing a simple concept but unfortunately missing…
We cannot tell which numbers are divisible by 3
For a number to be divisible by 3, we must know the sum of ALL digits
I am not sure what you mean by option A being divisible by 3
Can you explain why you think option A is divisible by 3?
Out of all the 5 options however, 4 are not divisible by 4
So we have to assume that once the two blotted out digits of option E are visible
The sum of integers of option E would become divisible by 3
Oh sorry, I thought we just need to sum only last 2 digits to find the divisibility of 4. I think now I got it. Question is what could be the answer, so we’re juat guessing, right? Among all of the factors of 24, we can only test 2 and 4, 2 works for all but 24 works only for the option E. So, we can assume that it might be the multiple of 24, am I right?
Yes you are correct