Arithmetic Quiz 1

GRE Quant Problem Solving
1

Hello,

  1. could you please explain how did you solve this? I entirely didn’t understand this :
    Ask-11

  2. Also, why tenth is false in this question? secondly, why the answer is C? how these two are the same as “3.4” ??? because 3.498 , because of 8, it becomes : 3.5 !:

    Ask-12
    Ask-12750×405 80.6 KB

2

Could someone please help me with these issues?

3

What is one possible value for a and b?

Not sure what you mean - did you watch the full video? He says “ignore” after all.

4

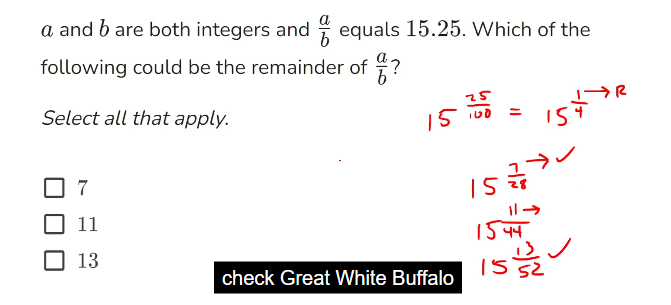
for the first one, one possible value can be considered : 1525 / 100 - is it right? a= 1525 , b = 100

5

He mentioned “ignore,” but earlier he stated that “tenth” was incorrect and it should be “hundredths”, I think. This comment doesn’t relate to the “ignore” directive! Additionally, he didn’t explain why these two could be considered equal. I was uncertain whether the question was wrong, but since he didn’t explicitly say it was, I believe there must be an answer to it.

6

The question in the quiz is not the same as that in the video - the difference is subtle but changes the answer.

7

Ok, what about the first question? you told me to make a possible example for a & b, but still I don’t understand it.

8

That is one possible value indeed. What is the smallest possible positive value of a and b?

9

If we simplify that value we can reach 61/4 ? is it right?

10

That’s one value. Can you now get the answer?

11

Unfortunately no…
Why in the video he says 7/28 is ok, 11/44 is ok , … without further explanation? could you please explain it to me more clearly?

12

Because \frac{7}{28} = \frac{11}{44} = \frac{13}{52}. In other words, we are using this to find possible remainders and check whether that matches the options.

13

How can we initially determine 25/100 to discover that 7, 11, and 13 can also fit? We could also state that 25/26, for instance, has a remainder of 25.

14

Could, where k is an integer,

k \frac{25}{26} = 15.25
15

I’m sorry I didn’t understand your reply. Still can’t figure out how we got to 25/100 from 15.25!

16

Put it this way: I asked whether we can write \frac{25}{26} as 0.25 - and the answer to this is no, because well that isn’t the case.

The idea of this question is showing that

0.25 = \frac{1}{4} = \frac{25}{100}

and hence

15.25 = 15 \frac{1}{4} = 15 \frac{25}{100}

Notice the multiples?

1 Like
17

oh I got it! THANK YOU SO MUCH!