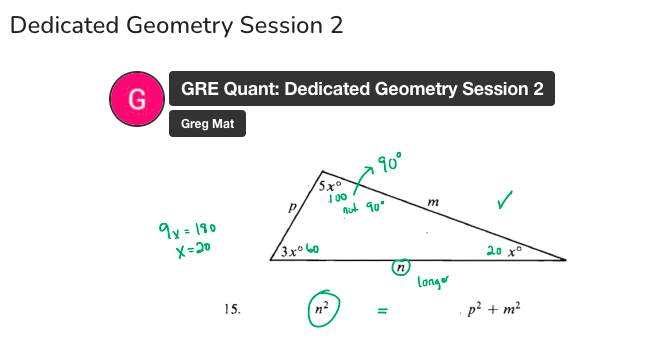
Hi guys, in the screenshot you can see the assignment from one of the recorded videos on gregmat (title visible). So what I used is a concept that states that the sum of two sides of a triangle is always longer than one other side. So p+m > n and therefore p^2+m^2 > n^2 but the answer is that n^2 is the biggest and I do not understand.
squaring on both sides will result in the identity (a+b)^2 = a^2+b^2+2ab for p+m part
i don’t understand this
If u substitute numbers for p, l & m and then compare it u wud realize that p^2 + m^2 would not be greater than n^2
p+m > n
squaring on both sides
(p+m)^2 > n^2
p^2+m^2+2pm >n^2
Ok I understand the identities now. Thank you
But my question also concerns the concept that 2 sides added together are larger than one other side. Is that not applicable or am I misunderstanding something there. Otherwise we would be breaking that rule, right?
This itself is not correct. Let p = 0.3, q = 0.4 and n = 0.6. p + q > n but p2 + q2 < n2.
This is correct, and you can indeed infer that p + m > n. But that is about it.