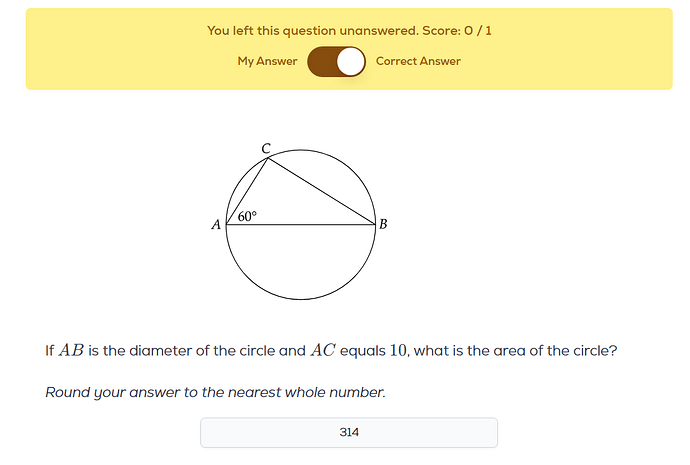
Hello, could someone explain how to solve this problem? I was able to create a 30 60 90 triangle by drawing a perpendicular line from point A to line segment AB. This allowed me to figure out the height of the triangle. When solving for the remainder of the base (segment AB), I got stuck; we do not know the rest of the interior angles of the second / right half of the divided triangle aside from a 90 degree angle.
Is there some rule about inscribed angles / triangles that I am missing? How can you solve this problem?
Thank you in advance for your help!