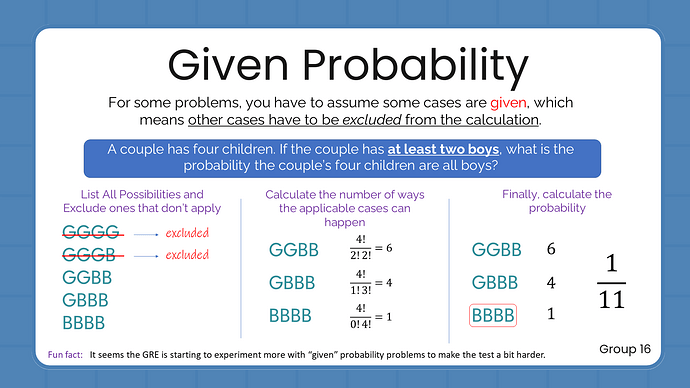
Hello g.
I am seriously confused as to why order matters in this problem. I think, as per the question statement, GGBB should be no different for the couple than BBGG.
If we think like that, the answer to this problem becomes 1/3. Am I correct?
In any case, how do we know whether the order of the children matters for the couple?
This is correct. However: the probability of a GGBB (order invariant) is more than GBBB (again order invariant).
Thanks for the answer.
I still have a confusion.
Aren’t the probabilities different only because order matters here?
My confusion is this: why does the order even matter here?
To put it another way, if a couple is begetting one girl and three boys, why do they care if they get GBBB, BGBB, BBGB, or BBBG?
The order per se doesn’t. What matters is the probability of getting GGBB for instance (i.e, two girls and two boys).
Umm…Sorry if I sound like splitting hairs here. But doesn’t the probability of getting a GGBB more because it bears more “arrangements” than, say, “BBBB?”
@gregmat
AAaaaah…I got it…I just compared it to a coin toss problem. Now I don’t understand why I couldn’t get it earlier. Strange.
Thanks for bearing with me.