GRE Big Book - Test 11, Section 1, Question 19

GRE Quant Problem Solving
1

Hi @gregmat,

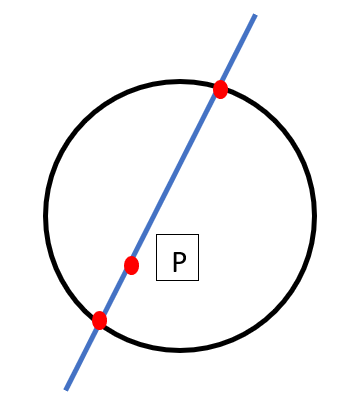
Question: C is a circle, L is a line, and P is a point on the line L. If C. L, and P are in the same plane and P is inside C, how many points do C and L have in common?

Answer: 2.

In this question, why it is assumed that line L crosses circle C completely (two points in common between C and L)? Wouldn’t be possible for line L to be discontinued after it reaches point P (one common point between C and L? The question does not specify a “Must” or a “Could”. Thanks for your help.

2

I think because a “line” extends infinitely in both directions whereas a “line segment” doesn’t

3

Thanks! I was really confused

4

As its stated that Point P is inside the circle (not on circle i.e. circumference), then it should have 3 points in common. One is point P and another 2 is, where Line L intersects circle. Could you please explain. I got confused with this question.

image