GRE Quant Question (Standard Deviation)

GRE Quant Problem Solving
1

Hi Guys,
Can anyone please tell me an easy way to solve this numerical

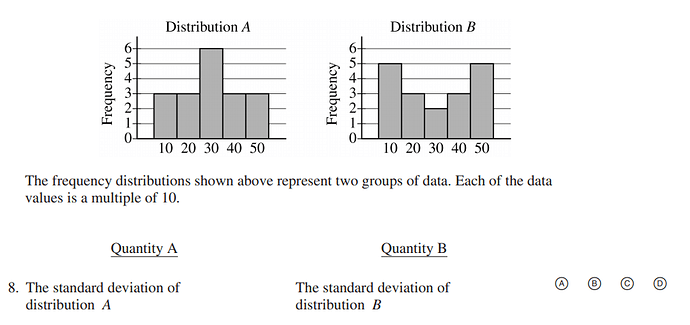
image
image930×439 50.3 KB

2

In this question, since the values at x axis have the same range, and the average number is 30 for both, we can simply look at the total distances of these 5 numbers to 30 in Q_{A} and Q_B. From the pics shown above, apparently, Q_ A is greater.
Edit: my mistake here, please refer to other nice relpies :slightly_smiling_face:

3

I would rather say that the standard deviation of B is greater than the one of A. It is true that for both groups the average is 30 (since both are simmetrically distributed). Now, you have to look for which group the spread is bigger from the average. In group A many numbers fall right into the average and not to many into the extremes. For B it is exactly the opposite. That’s why the standard deviation of B is bigger than the one of A.

4

What does Q denote here?

5

This is a good way, but I am not sure if I could confidently use this method in the actual exam.

6

quantity

7

To solve this intuitively you need to first need to have an idea of couple of things: Symmetric distribution , what is SD ?

If you are able to notice that both graph are symmetrically distributed then : Mean = Median = 30

Now , SD is nothing but how spread out numbers are from mean . Comparing Distribution A to B we see that values are much more spread in Dist. B than in A. Hence , Qty B > Qty A

Also read this much more detailed solution at greprepclub : https://greprepclub.com/forum/the-frequency-distributions-shown-above-represent-two-groups-8090.html

1 Like
8

After finding the Mean, why don’t you just rewrite the observations for both. (this may work for this example only as the observations are less)

10,10,10,20,20,20, 30,30,30,30,30,30, 40,40,40,50,50,50

10,10,10,10,10,20,20,20, 30,30, 40,40,40,50,50,50,50,50

Look at the spread now. In A more observations are near to mean. and less are the outliers
So A is smaller than B (as while calculating SD, those observations equal to mean will become 0)

1 Like
9

This is a good way to do it and a safe approach! I made a mistake when the first time I saw this. Good clarification!