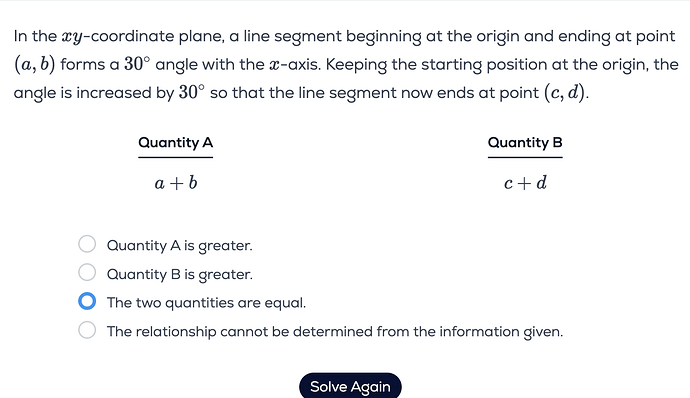
Hi,
Looking at this question:
In the question, no where does it state that (a,b) are positive. Suppose a<0 and b>0 such that a^2 + b^2 = 2.
a = -\sqrt{3} , b= 1 satisfies this and a line from the origin to (a,b) will satisfy a 30^{\circ} angle with the x-axis. If we increase this angle to 60^{\circ} as it states in the question, (c,d) = (-1,\sqrt{3}).
Therefore,
a+b = 1-\sqrt{3} \neq c+d = \sqrt{3} -1
And I chose D.
Is this a mistake in the question or am I missing something?
Thanks.
In coordinate geometry, i guess points are stated as (a,b) for positive and (-a,-b) for negative. We just need to plug value as given if required. In your case, as (a,b) is given, you are just required to consider it as positive only.Am i correct @gregmat ?
No; the OP is correct. If the coordinates must be positive, this must be stated explicitly.
This question is faulty, and we’ll get this repaired. @gregmat
To the OP: which quiz is this from (if this is from the problem-solving section, what’s the title of the question)?
@colecitrenbaum Can you please answer my question above? We need that to fix the error.
Sorry for the delay. It took me a while to find this again. This is a “hard” QC question from the problems/quant page. The video is quant_854 and the text begins with “in the xy-coordinate plane…” as in the picture.
That error has been fixed.