Intuition for maximizing volume of cylinder inside box?

GRE Quant Problem Solving
1

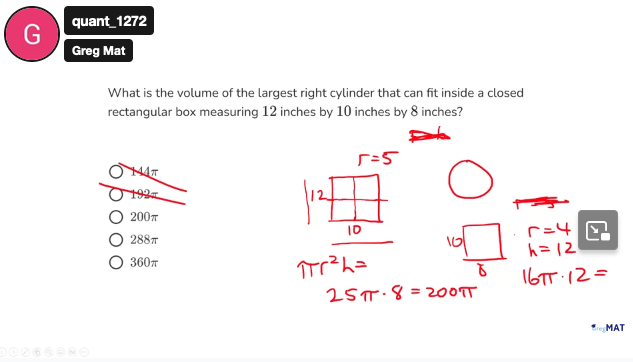
The solution video only considers the case where the cylinder is flush to one side such that its height is equal to 8, 10, or 12. But what about the diagonal, which will be longer per the Pythagorean theorem?

I tried working out the algebra for the diagonal case, but it got complicated very quickly. I assume the solution video is correct, so I abandoned this.

But here is my question: how do we know the flush case results in the maximum volume for the cylinder? Is there a quick, intuitive way to determine why flush is better than diagonal?

I am trying to develop my mathematical intuition for the GRE so I can avoid rabbit holes like the aforementioned complex algebra.

Thank you!

0

2

What diagonal? You want to place the cylinder along the space diagonal of the rectangular box or do you mean something else?

3

Imagine the ruler was a cylinder that actually fit inside the box. By “diagonal,” I mean like this photo. Does that make more sense?

0
0553×741 46.5 KB