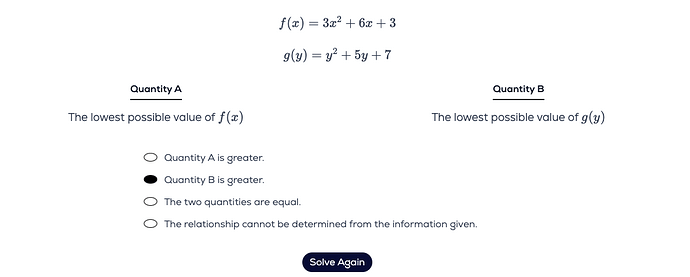
I approached this question a little differently, I used the discriminant to figure out that the value of f(x) would be zero since the discriminant is zero in Quantity A and g(y) would be positive in Quantity B since the discriminant is less than zero. I am wondering if this makes sense too
Okay so Discriminant being zero doesn’t necessarily mean that the minimum value of that function will be zero. If the curve is facing downwards and Discriminant = 0, zero is actually the maximum value in this case. In y = ax^2 + bx + c, if a is positive, then graph is facing upwards ( in the above question, both are facing upwards since a is positive in both cases). Now considering these upward facing graphs, if Discriminant =0, then zero is the minima. If Discriminant was greater than zero, then the minima would be negative ( 2 roots). If Discriminant is less than zero, the minima is positive ( no roots).
So if you considered the fact that both graphs were facing upwards, you did fine.
I had assumed that both of these curves were facing downwards, thanks for pointing it out.