Could anyone please explain the solution to this problem? I’m unable to understand the one given in the book.
Thanks in advance!
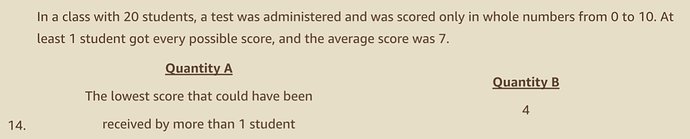
Give : 1 student got every possible score ranging from 0-10(Whole Number)
Avg. = 7
Student 1,Student 2…, Student 11 = (0,1…,10)
Thus, 11 Student in total got 55 marks
Now; using average : \frac{x}{20} = 7 where; x = Sum of all observations thus, 20 \times 7 = 140
140 - 55 = 85( 9 Children need to get this) Thus, maximizing the sum should give us the minimum score:
take 8 children each score 10 thus remaining one will score 5; ANS = 5
Could you please explain why we take 11 students?
I’m unable to understand this part too; why do we subtract the 2 sums and consider 9 children?
Student No Score
Student 1 = 0
Student 2 = 1
Student 3 = 2
Student 4 = 3
Student 5 = 4
Student 6 = 5
.
.
.
.Student 11 = 10
The formula for average is \text{Average} = \frac{\text{Sum of all observation}}{\text{Total Number of Observation}}
Now, question already give us the average(which is 7) and we know that the total number for observation (20 because we have 20 student); thus we can calculate: Sum of all observation which is 7x20 or 140. Now, we know that atleast 1 student got every possible score (range is 0-10 i.e. 11 numbers) thus; we subtract the sum of that (55) from 140 to get 85.
Oh, I think I get it now
- So we consider the first 11 students’ score as 0-10 to satisfy the criteria of “every possible score”.
- Next we get the score for remaining students (10-11 = 9 students) which is -
140 (total of 20 students) - 55 (score of the first 11 students, sum of 0-10) - Then we maximize 8 of the scores so that the last student has the least score - which would be 5.
It finally makes sense! Thanks for the in depth explanation!