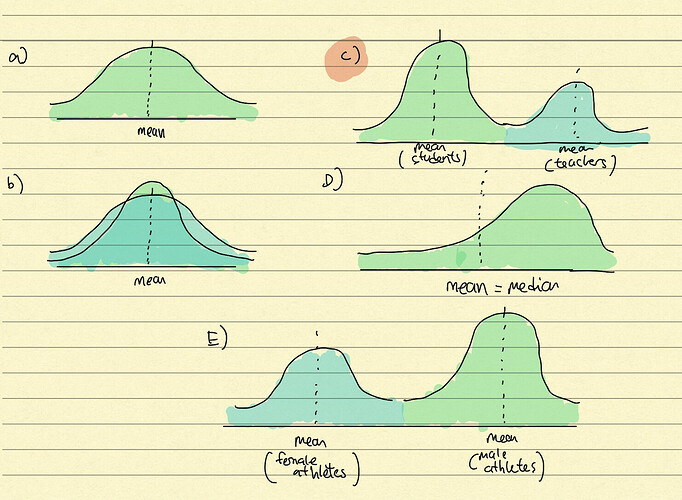
As you can see A, B and D are nothing like the image.
D is wrong because it says the small data set (female athletes) has a lower mean, therefore the peak of the left has to be shorter. But the image in the question shows the peak of the left being taller.
Note: mean goes from low to high from left to right.
By POE, C is correct. While answer C does not specify which peak has a lower mean, it is the only answer that isn’t a mismatch solely based on its description.
Does smaller data set always have lower peak than the bigger data set?
And to check, while skewing to the left, median is greater than mean? Isn’t it?
My bad on D. You are correct, for a left skewed distribution, the median > mean.
The peak (or height) of a normal distribution = the frequency/number of observations. The lower the number the lower the height (as compared to another dataset with higher frequency of observation)
As an analogy, you can think of the bell curve as a sand mound, the more sand (datapoint) you have, the higher you can pile up the sand mound.