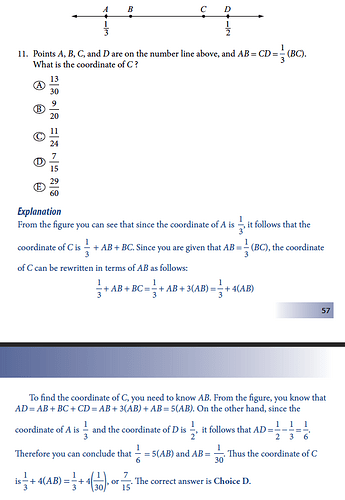
I am quite stuck with this question - can someone lend a helping hand to untangle me?
I can follow the logic of the solution except when they say A=1/3 (which is given) and it follows that C = 1/3 + AB + BC. What’s the relationship between the two and more importantly, how do I get to C = 1/3 + AB + BC?
Imagine you’re walking along a number line. You start at point A, which is at 1/3. You know that point D is at 1/2. Now, you’re told that there are two more points, B and C, between A and D. The distances between these points have a special relationship: the distance from A to B is the same as the distance from C to D, and both of these distances are one-third of the distance from B to C.
So, let’s think about this in terms of steps. If we say that the distance from A to B is one step, then the distance from B to C must be three steps (because it’s three times the distance of AB). And since CD equals AB, the distance from C to D is also one step.
So, in total, from A to D (which we know is a distance of 1/2 - 1/3 = 1/6 on the number line), we have 1 (AB) + 3 (BC) + 1 (CD) = 5 equal steps. Each step, therefore, is of length (1/6)/5 = 1/30.
Since point C is at a distance of 1 (AB) + 3 (BC) = 4 steps from point A, and each step is of length 1/30, the coordinate of point C is at A + 4*(1/30) = 1/3 + 4/30 = 7/15.
p.s. Feel free to ping if you’re still having anymore doubts
