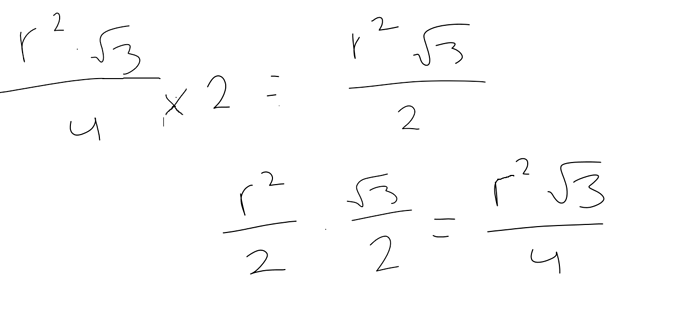Hey y’all, this may be a silly question but I have been staring at this for a long time trying to figure out where my math went wrong.
The question is trying to get you to calculate the area of two equilateral triangles with side length r. I used Greg’s (half * half * root3) formula to get [(r^2 * root3) / 4]. When I multiply this by 2, I get [(r^2* root3) / 2]. The correct answer is (root3)/2 * r^2.
Where I am getting confused is I thought I would be able to split my answer into two terms, each over 2. So I would have r^2 / 2 and root3 /2. But clearly this is not right and I can’t find a reason why online. I don’t understand why the answer only places the 2 in the denominator under the root3 and not the r^2.
Any thoughts?
They are the same though?
I guess where I am getting confused is that I would have thought if you split up the terms in the numerator, you would have two fractions: one that is r^2 / 2 and one that is root3 / 2. But then if you multiplied that together, the answer then has a 4 in the denominator… would that then simplify?
Such a silly question but it has been plaguing me
I am still confused.
Yes, but then you would multiply the fraction by 2, making the denominator 2…
Maybe this helps explain where my issue is better if you can see the math.
Once I multiply the area of one triangle [(r^2 * root3) / 4] by 2, I get [(r^2 * root3) / 2].
If I then split the fraction to each have a denominator of two, when I re-combine I end up exactly where I was before? Where I am getting confused is I don’t understand how the answer choice only has a denominator of 2 under the the root3 and not the under the r^2… so is it that in this case I would NOT put a 2 under both fractions but rather one of them would need to be a 1?
This is not correct:
\frac{r^2 \sqrt{3}}{2} \neq \frac{r^2}{2} \times \frac{\sqrt{3}}{2}
Is that what you’re asking with “split the fraction to each have a denominator of two”?
Yes - thank you for the clear visual. I didn’t know that wasn’t “allowed.”
So then how do I get from what you have on the left to the right answer which is r^2 * (root3 / 2)? I guess where my confusion lies is if I cannot split the fraction (as you showed above) then how can I only put the denominator of 2 under the root 3 and not r^2?
I know this is probably a very elementary question manifesting in a complex problem, so I appreciate your patience 
Split the components of the fraction. You have three:
In
\frac{r^2 \sqrt{3}}{2}
notice that you’re just multiplying the above three components.
This is the same case in
\frac{\sqrt{3}}{2} \times r^2
After all, \frac{\sqrt{3}}{2} is the same as multiplying \frac{\sqrt{3}}{2} and \frac{1}{2} - does this help?
When you’re splitting \frac{r^2}{2} \times \frac{\sqrt{3}}{2} though, you have two instances of \frac{1}{2} that are being multiplied, not 1. Notice that
\frac{r^2}{2} \times \frac{\sqrt{3}}{2} = \frac{1}{2} \times r^2 \times \sqrt{3} \times \frac{1}{2}
i.e, you have four components, not three.
This was so helpful - consider me enlightened.
Thank you!!!