The solution video suggests that the prime factors may have exponents other than 1, e.g., (2^2)(3^1)(5^1). I thought “distinct” implies each prime has an exponent of 1. If it does not, what exactly does “distinct” mean here? Thank you!
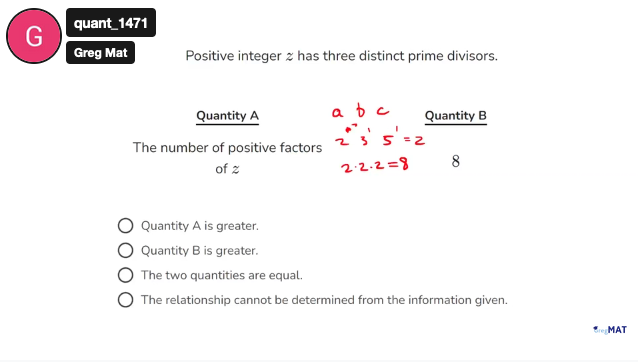
Let n = 2^3 \cdot 5^8 \cdot 7^2
This number has 3 distinct prime factors because well among all the divisors only 3 are prime. Those 3 prime divisors are 2, 5, and 7. Nothing is restricting the 2,5, or 7 from having an exponent greater than 1 because regardless of the exponent on all 3 (obviously excluding integers that aren’t in range), you’re not changing the number of prime divisors.