QC question from Greg's Quant Concept Practice

GRE Quant Problem Solving
1

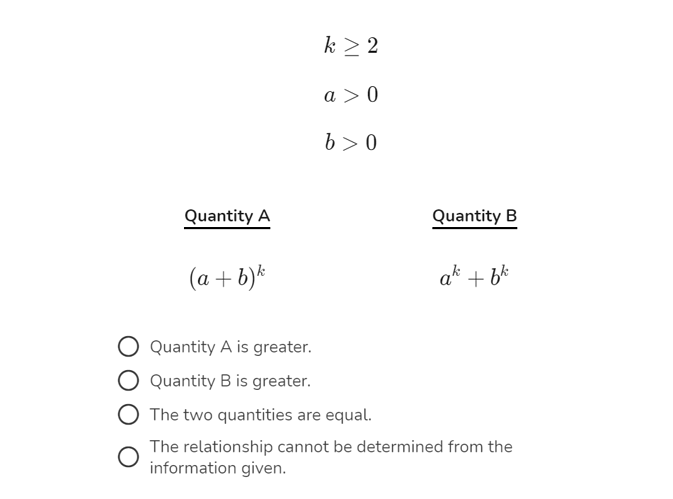
This is an easy QC problem from Greg’s quant concept practice on the website.

image
image951×706 15.9 KB

k is greater than or equal to 2.
a> 0, b> 0

Qty A: (a+b)^k
Qty B: a^k +b^k

The answer is A, because in the video explanation Greg says since the ‘2ab’ factor is always present in Qty A, it’ll always be bigger.

My question is I tried a fractional power for a and b because the question only says they’re positive and not necessarily integers. I did a=1/2, b=1/3 and in this case Qty A is (root) 3 ~ 1.732 and Qty B is (root)1 + (root) 2 ~ 1 + 1.414 = 2.414 and Qty B is bigger, so I chose D.

2

I do not get how you got that. If a = \frac{1}{2} and b = \frac{1}{3}, quantity A is {(a + b)}^k = \left(\frac{5}{6}\right)^k, and I do not see \sqrt{3} anywhere.