amartya
1
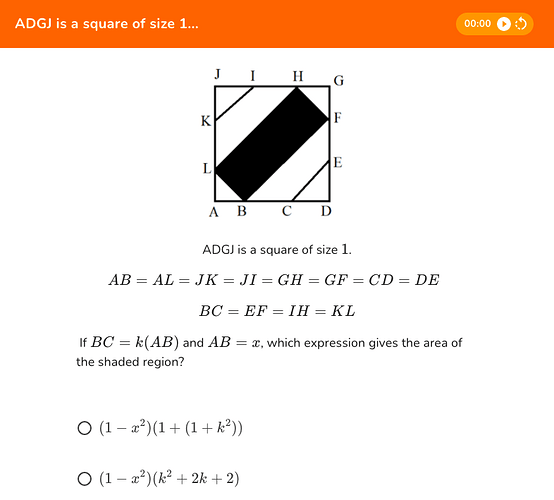
Hi, I solved it like:
Given, AB=AL=x , hence LB = √2 x (since 45-45-90 triangle)
Also, (BC+CD)=(EF+ED) = kx+x, hence BF= √2 (kx+x) (since 45-45-90 triangle)
We can prove the shaded figure is rectange, so
area =
lengthbreadth = LBBF = 2x(kx+x)
But the answer is different, where am I wrong? (PS: I have seen Greg’s method, he’s using numbers to do it but here I wanna try the algebraic method)
Your answer is not wrong the answer just expects you to solve it differently
If you wish to algebraically obtain it, then
Area of entire square = 1
Area of BDF = area of LJH = \frac{(kx + x)^2}{2}
area of LAB = area of HGF = \frac{x^2}{2}
area of shaded region = 1 - 2*\frac{(kx + x)^2}{2} - 2*\frac{x^2}{2}
= 1 - (kx + x)^2 - x^2
= 1 - k^2x^2 - x^2 -2kx^2 - x^2
= 1 - k^2x^2 - 2x^2 -2kx^2
= 1 - x^2(k^2 + 2 + 2k)
= 1 - x^2(1 + (k + 1)^2)