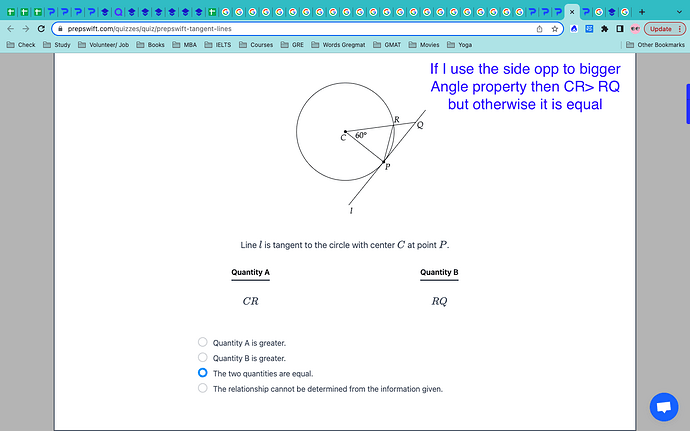
In this question, I believe, CR can be greater than RQ if we use the angles side property, which makes the answer D.
You’ll need to explain further.

I will probably solved it this way (Don’t know the solution so correct me if I am wrong!)
As In triangle CPQ rt. angled at P → angle C = 60 ; Angle P = 90 and Angle Q = 30 (180 - 90 -60 = 30)
Now, in Triangle CPR : CP = CR (radii of the circle) and Angle C = 60 .
We know that angle opposite to equal sides are also equal thus, let the Angle P be x hence, Angle R will also become x. Now, x +x+60 = 180 or x=60 → Triangle CPR is equilateral!
Now , in Triangle RPQ : Angle Q = 60 , Angle P = 30 (90 - 60= 30) and Angle R = 90 (180 - 30 - 60 = Angle R).
Now, RQ is opposite to angle 30 while CR is opposite to angle 60 and we know that side opposite to the larger (greater) angle is longer.
But that’s two different triangles…
Got it. Even I was considering both the triangles to compare.