Any other solution to this please?
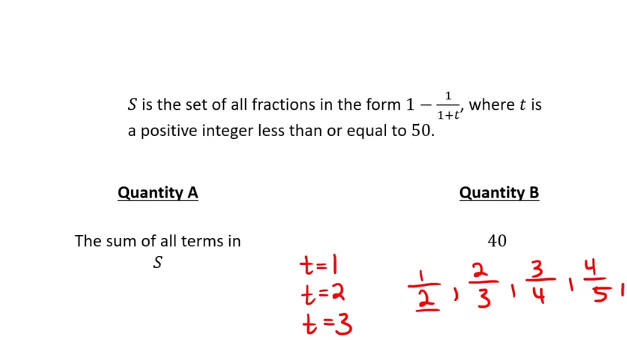
Apologies if my handwriting’s too small.
You could do this through harmonic progression. But imo its overkill, the formula’s a pain in the butt. In addition, they’re not asking the exact value, just a comparison, so an approximation would suffice.
Hope this helps.
2 Likes
This is not doable in a timed test.
It is. The solution might look big. But I did this problem in under 2 min in my rough. And imo this is in the medium-hard territory. So 95-110 second should be fine. Assuming you save at least 20-30 seconds for every easy question.
Like it or not, I recommend that try to get the hang of the method Greg’s taught in the video solution. I saw lots of people with high scores solve similair GMAT sequence questions by implementing the method Greg taught, which almost like an official approach lol. Choosing a representative number in sequence then assume the rest has the same value, multiply with a number and compare based on the context of the question.
The following is the estimate value of the sum of this sequence by setting up an integral.
\qquad \int_{1}^{50}\left(1-\frac{1}{1+t}\right)dt=\left[t-\ln(1+t)\right]_{1}^{50}
\qquad=49-\ln\left(\frac{51}{2}\right)\approx45.76
I just realized that the GRE calculator doesn’t contain logarithm function, which means if you don’t know how to estimate the approx solution of log function the problem still stands, so again, Greg’s method is preferable.
1 Like
@Leaderboard, you … 
The smallest quantity in the set is 0.5
So their total sum would be larger than 0.5 * 50 = 25
Now that you have taken 0.5 from all numbers, the smallest number you are left with is 0.67 - 0.5 = 0.17
The total sum of these remaining numbers hast to be greater than 0.17 * 49 = 8.33
8.33 + 25 = 33.33
Now that you have taken 0.5 + 0.17 = 0.67 from all numbers, the smallest number you are left with is 0.75 - 0.67 = 0.08
The total sum of these remaining numbers hast to be greater than 0.08 * 48 = 3.84
33.33 + 3.84 = 37.17
Now that you have taken 0.5 + 0.17 + 0.08 = 0.75 from all numbers, the smallest number you are left with is 0.8 - 0.75 = 0.05
The total sum of these remaining numbers hast to be greater than 0.05 * 47 = 2.35
37.17 + 2.35 = 39.52
You can obviously do this step another time, but to me reaching here is obvious enough that A > B
2 Likes
@chloel97 an integral would imply that it includes non integral values also right? It is approximately the same in this case, because the graph quickly flattens, but I doubt we can interchange summation and integrals for all equations.
See the Euler-Mascheroni constant.
Chloe: integrals are beautiful, so I just adjusted the latex for that.
1 Like
I agree with Shrey that you have to find the trend and not like the exact solution…if you get the graph you can see that the HP series can never surpass the value of 10 as higher the denominator goes the lesser the reciprocal gets.
Hence the answer is A.
Appologies for the handwriting
We certainly can’t. First of all, the function we choose to integrate has to be a continuous function, like what we saw in this case. Secondly, not all continuous functions can be integrated, this is proved by Risch Algorithm.
You may not be able to “interchange” them verbatim, but there is a good case for replacing an integral with summation and vice-versa in some cases as long as you know what you’re doing.
After all, a key concept of Riemann integration are upper and lower sums, which would be simply a summation over a dissected region into n equal parts.
Anyway, from a GRE point of view I agree that Greg’s method is preferable.
Thanks everyone.
Could somebody plz point me to the Greg’s method to solve this one?
See the video solution attached to that question (https://www.gregmat.com/problems/problem/s-is-the-set-of-all-fractions-in-the-form/)
1 Like