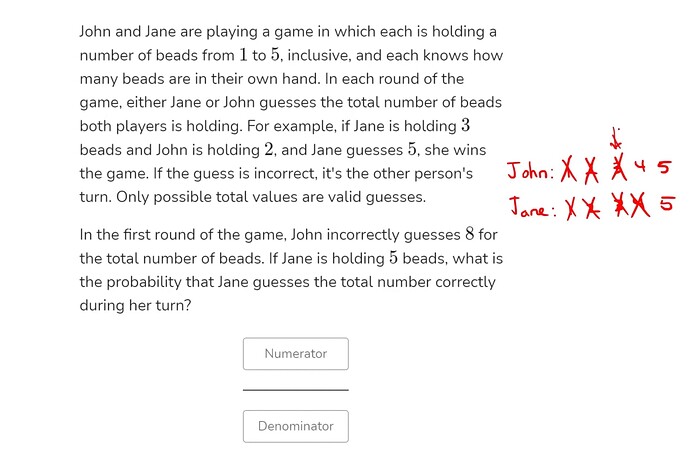
i understand cancelling 3 from John, but we only know 8 is incorrect. this doesn’t make a total of 6[5+1], or 7[5+2] incorrect though? Where is it mentioned that the other person can only guess upwards?
then the answer should be 1/4 [John’s 1,2,4,5] ?
We assume that Jane is a logical person. If John guessed an 8, then this assumes that John at least chose a 3, no? For instance, if John had a 1 then he wouldn’t choose something like 8 because it’s impossible.
but we’re actually free to make assumptions this way on the exam? like this I understand, but I want to ensure I don’t end up making a mistake like this in the test
Uh, i just assumed from context. You don’t have to assume they’re logical individuals though, but i guess that would stray away from the intent of the question. As long as your inference/deduction is a sound one then I wouldn’t worry too much about what shows up on the exam.
Where is this question from? Is it part of a quiz or …?
This needs a closer look, because arguments about people being “logical” works in a job interview but not in a GRE test (even though yes one should not make “unreasonable” assumptions, I need to see the solution video to comment further).
GRE quant problem solving question on the website, data analysis extreme
just wanted to find if there was any update on this. does 1/2 still hold, or is 1/4 correct?
Looking at this further: when John plays the game, he knows how many beads he has right now. So when he says 8, this means that Jane has 3, 4 or 5 beads from John’s perspective - and Jane can deduce that John has at least 3 marbles in turn. On those lines I think the question is OK.
Is this a reasonable question? Hard to say, because this kind of logic is kind of beyond what one would see on the GRE. However, I cannot pinpoint a good reason to declare this question as faulty either.