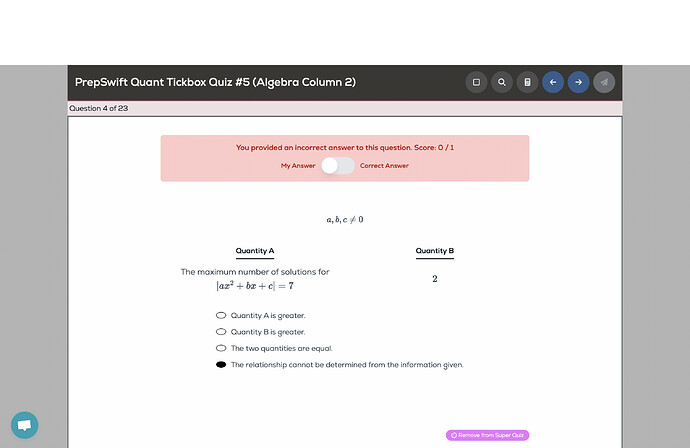
Hey guys, I had a doubt in this question, since we don’t know anything about a,b,c except that they are not equal to zero, how do we determine whether the determinant is going to be positive or negative?
I think the correct answer is A.
Because:
|ax^2 + bx + c| = 7 can be broken into two separate quadratic equations:
ax^2 + bx + c = 7 or ax^2 + bx + c = -7
Depending on its discriminant, each quadratic equation can have 0, 1, or 2 real solutions. Therefore, the maximum number of solutions for the equation ax2 + bx + c = 7 is 2. Similarly, ax^2 + bx + c = -7 could have a maximum of 2 solutions.
Thus, the maximum number of solutions for the equation |ax^2 + bx + c| = 7 is 4.
Thank you Cindy! I understand the question now, potential maximum answers is always 2 for a quadratic equation. Thanks!
Hi Cindy, thank you for your response.
I would appreciate a clarification if possible.
Since we determine the number of solutions by looking at the discriminant, I implied that because we had no information on the relationship between (c - 7) and b^2/4a or (c + 7) and b^2/4a we could not determine the precise number of solutions.
So, does the correct answer to this question just come down to the word “maximum”? In other words, every time they state “the maximum number” should we take the best-case scenario no matter what?