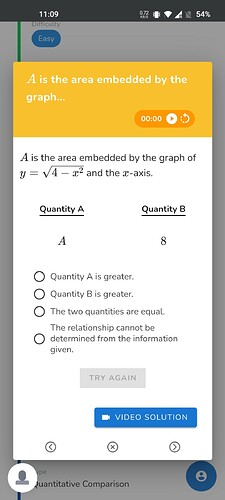
Hello ,
In this question why can we never have y as (-)ve value? (As explained in the video solution)
If we write the expression as y = [(-2)^2 - x^2]^1/2
In this case , if we substitute x = 0 , then we can get
“y = -2”
So we can consider the full circle area right?
Can anyone please clarify this doubt?
Thank you
Suggestion: please take a screenshot or link to the problem as this is very hard to read. But no, it’s not a full circle.
Hello , I have uploaded a clearer screenshot .
Also , can you please explain why y = (-2) is not possible ?
Classic GRE rule: the root (on the RHS) is always positive. Also use Latex wherever needed.
But , a root of 4 can be either +2 or -2 no?
The value of y in y = \sqrt{x} is always nonnegative.
1 Like
So , in a typical GRE question , I can never consider the case 2 ?
Can you please confirm ( Sorry for repeated questions)
Thanks!
Not in general. The problem is that \sqrt{x} must be nonnegative, which means that -a will fail.
1 Like
Ok thank you for the help