Why was x-3 multiplied by (x-a)^2

GRE Quant Problem Solving
1

image
I did not understand this step and feel that this hasnt been taught in any of the videos
Also pls tell in which scenario we will multiply by (x+a)^2

2

Do you know how to take out common ?

The easiest way to takeout common term from the polynomial equation is do polynomial long division:

x^2 - 4x + 4
______________________
x - 3 | x^3 - 7x^2 + 16x - 12
        - (x^3 - 3x^2)
        -------------
              -4x^2 + 16x
              - (-4x^2 + 12x)
              --------------
                      4x - 12
                      - (4x - 12)
                      -----------
                            0

so, if we know that 3 is one of the root of equation y = x^3 -7x^2+16x+12 and we also know that this equation will exactly have one more root then taking (x-3) common from y = x^3 -7x^2+16x+12 will give x^2 - 4x + 4

Therefore, we can rewrite y = x^3 -7x^2+16x+12 as (x-3) (x^2 - 4x + 4) and further (x^2 - 4x + 4) can be written as (x-2)^2 thus, overall equation becomes (x-3) (x-2)^2

3

idk long division so I hope this type of problem doesnt show up on gre

4

It’s one of the various concepts that we learn as a part of our high school math curriculum and most of us have forgotten it by the time we start prepping for GRE but basic concepts like this are often helpful to understand various math concept tested by ETS. Also, roots are an important topic under ALGEBRA and PowerPrepOne already has one question related to this concept(concept of roots). So, this concept is definitely important !!

Spoiler warning: PP1 root question : https://www.prepscholar.com/gre/blog/one-roots-equation-x2-kx-6-0-3-k/

5

I dont understand what u r trying to imply perhaps the link to the question that u shared only requires us to put the value of x and solve for k
it doesnt involve long division!
both questions are different on a complete different level!

6

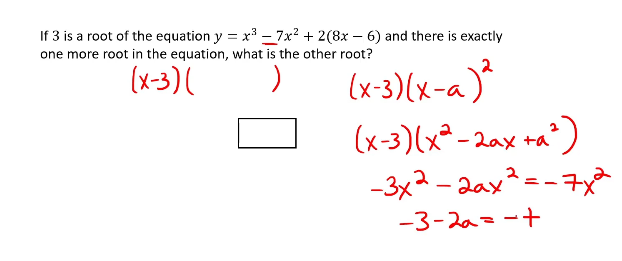
Notice that the question says that

  • 3 is a root - (x - 3) divides y
  • there is exactly one more root - call it a

The latter means that one of the roots must be repeating. So either we have

  • y = (x - 3)^2 (x - a) - with 3 repeating
  • or y = (x - 3)(x - a)^2 - with a repeating

Greg is checking the second case by expanding it. Once the expansion is done, we can simply find a such that it’s equal to the original equation given in the question.

Long division is possible, but is not required for this question. In fact, it wasn’t even intended - because otherwise, you don’t even need the “exactly one more root” part.

7

Sorry, If I was not explicitly clear from what I meant by my earlier post. The question above just require to understand the concept of roots, long division is just one of the way to tackle the above question (not necessarily the only way) and you can solve the question even without it (see Leaderboard post below). And from the PP1 question that I said earlier , I was implying that roots of an equation is an important concept and is tested often at GRE so, don’t skip it!